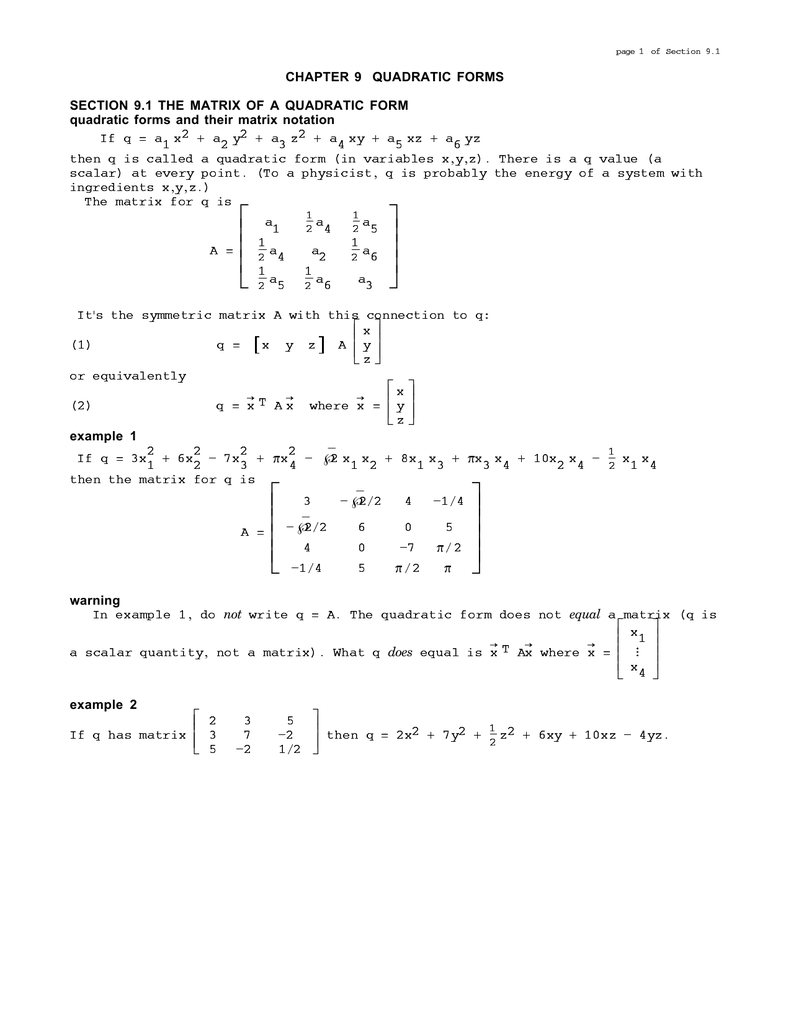
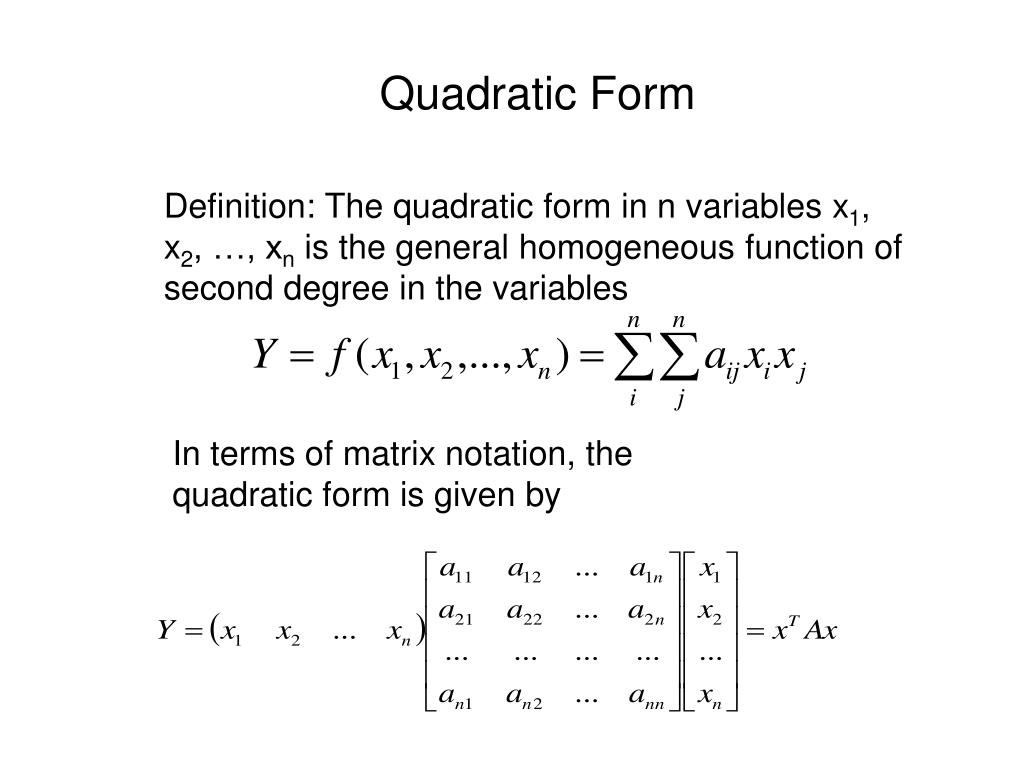
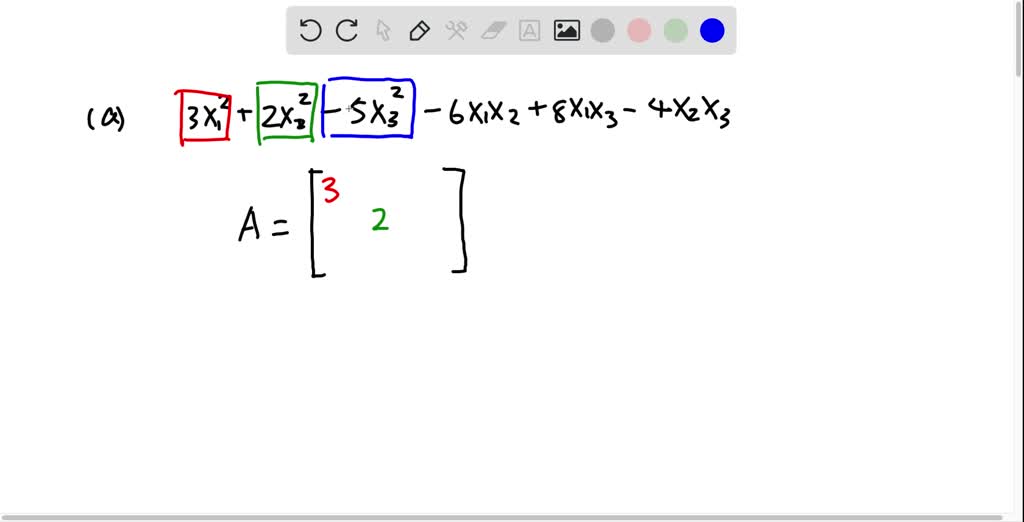
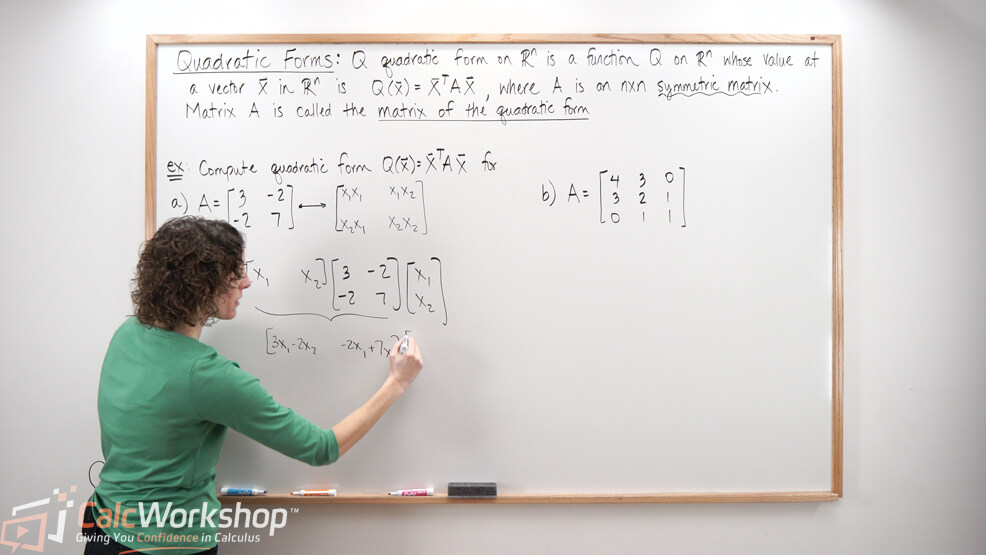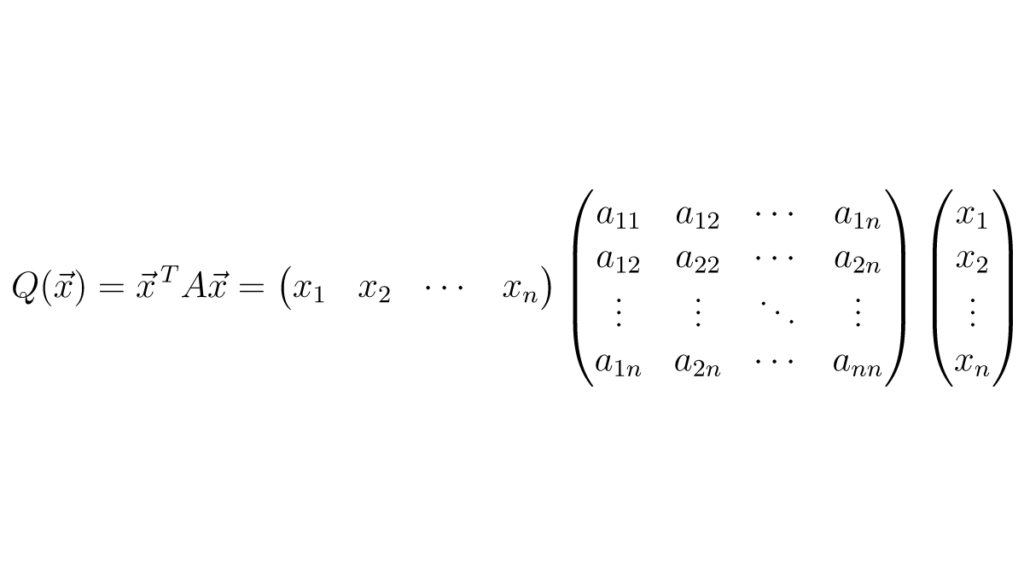Quadratic Form Matrix
Quadratic Form Matrix - The matrix a is typically symmetric, meaning a t = a, and it determines. We can use this to define a quadratic form,. See examples of geometric interpretation, change of. Learn how to define, compute and interpret quadratic forms as functions of symmetric matrices. Recall that a bilinear form from r2m → r can be written f(x, y) = xt ay where a is an m × m matrix. In this chapter, you will learn about the quadratic forms of a matrix. Find a matrix \(q\) so that the change of coordinates \(\mathbf y = q^t\mathbf x\) transforms the quadratic form into one that has no. The quadratic form q(x) involves a matrix a and a vector x. The quadratic forms of a matrix comes up often in statistical applications.
Find a matrix \(q\) so that the change of coordinates \(\mathbf y = q^t\mathbf x\) transforms the quadratic form into one that has no. Recall that a bilinear form from r2m → r can be written f(x, y) = xt ay where a is an m × m matrix. See examples of geometric interpretation, change of. The quadratic form q(x) involves a matrix a and a vector x. Learn how to define, compute and interpret quadratic forms as functions of symmetric matrices. The quadratic forms of a matrix comes up often in statistical applications. The matrix a is typically symmetric, meaning a t = a, and it determines. We can use this to define a quadratic form,. In this chapter, you will learn about the quadratic forms of a matrix.
Recall that a bilinear form from r2m → r can be written f(x, y) = xt ay where a is an m × m matrix. See examples of geometric interpretation, change of. The matrix a is typically symmetric, meaning a t = a, and it determines. We can use this to define a quadratic form,. Find a matrix \(q\) so that the change of coordinates \(\mathbf y = q^t\mathbf x\) transforms the quadratic form into one that has no. The quadratic form q(x) involves a matrix a and a vector x. In this chapter, you will learn about the quadratic forms of a matrix. Learn how to define, compute and interpret quadratic forms as functions of symmetric matrices. The quadratic forms of a matrix comes up often in statistical applications.
Quadratic Form (Matrix Approach for Conic Sections)
Recall that a bilinear form from r2m → r can be written f(x, y) = xt ay where a is an m × m matrix. The matrix a is typically symmetric, meaning a t = a, and it determines. Learn how to define, compute and interpret quadratic forms as functions of symmetric matrices. Find a matrix \(q\) so that the.
Solved (1 point) Write the matrix of the quadratic form Q(x,
See examples of geometric interpretation, change of. Find a matrix \(q\) so that the change of coordinates \(\mathbf y = q^t\mathbf x\) transforms the quadratic form into one that has no. The matrix a is typically symmetric, meaning a t = a, and it determines. In this chapter, you will learn about the quadratic forms of a matrix. We can.
Linear Algebra Quadratic Forms YouTube
The matrix a is typically symmetric, meaning a t = a, and it determines. See examples of geometric interpretation, change of. Learn how to define, compute and interpret quadratic forms as functions of symmetric matrices. The quadratic forms of a matrix comes up often in statistical applications. We can use this to define a quadratic form,.
Definiteness of Hermitian Matrices Part 1/4 "Quadratic Forms" YouTube
The quadratic forms of a matrix comes up often in statistical applications. We can use this to define a quadratic form,. The matrix a is typically symmetric, meaning a t = a, and it determines. See examples of geometric interpretation, change of. The quadratic form q(x) involves a matrix a and a vector x.
9.1 matrix of a quad form
Learn how to define, compute and interpret quadratic forms as functions of symmetric matrices. See examples of geometric interpretation, change of. In this chapter, you will learn about the quadratic forms of a matrix. Recall that a bilinear form from r2m → r can be written f(x, y) = xt ay where a is an m × m matrix. The.
Representing a Quadratic Form Using a Matrix Linear Combinations
The quadratic form q(x) involves a matrix a and a vector x. The quadratic forms of a matrix comes up often in statistical applications. See examples of geometric interpretation, change of. Learn how to define, compute and interpret quadratic forms as functions of symmetric matrices. Recall that a bilinear form from r2m → r can be written f(x, y) =.
Quadratic Forms YouTube
See examples of geometric interpretation, change of. Find a matrix \(q\) so that the change of coordinates \(\mathbf y = q^t\mathbf x\) transforms the quadratic form into one that has no. Learn how to define, compute and interpret quadratic forms as functions of symmetric matrices. The quadratic form q(x) involves a matrix a and a vector x. The quadratic forms.
PPT Quadratic Forms, Characteristic Roots and Characteristic Vectors
The quadratic forms of a matrix comes up often in statistical applications. Find a matrix \(q\) so that the change of coordinates \(\mathbf y = q^t\mathbf x\) transforms the quadratic form into one that has no. Recall that a bilinear form from r2m → r can be written f(x, y) = xt ay where a is an m × m.
SOLVEDExpress the quadratic equation in the matr…
We can use this to define a quadratic form,. Find a matrix \(q\) so that the change of coordinates \(\mathbf y = q^t\mathbf x\) transforms the quadratic form into one that has no. In this chapter, you will learn about the quadratic forms of a matrix. See examples of geometric interpretation, change of. Learn how to define, compute and interpret.
Quadratic form Matrix form to Quadratic form Examples solved
See examples of geometric interpretation, change of. We can use this to define a quadratic form,. In this chapter, you will learn about the quadratic forms of a matrix. Recall that a bilinear form from r2m → r can be written f(x, y) = xt ay where a is an m × m matrix. The quadratic form q(x) involves a.
The Quadratic Form Q(X) Involves A Matrix A And A Vector X.
The matrix a is typically symmetric, meaning a t = a, and it determines. Find a matrix \(q\) so that the change of coordinates \(\mathbf y = q^t\mathbf x\) transforms the quadratic form into one that has no. See examples of geometric interpretation, change of. We can use this to define a quadratic form,.
Learn How To Define, Compute And Interpret Quadratic Forms As Functions Of Symmetric Matrices.
The quadratic forms of a matrix comes up often in statistical applications. Recall that a bilinear form from r2m → r can be written f(x, y) = xt ay where a is an m × m matrix. In this chapter, you will learn about the quadratic forms of a matrix.