Operator Definition Math
Operator Definition Math - Operators take a function as an input and give a function as an output. An operator is a symbol, like +, ×, etc, that shows an operation. The difference between an operator and a function is simply that we've decided to call the operator an operator and we've decided to. A mapping of one set into another, each of which has a certain structure (defined by algebraic operations, a topology, or by an order. A term is either a single number or a. As an example, consider $\omega$, an operator on the set of functions. A symbol (such as , minus, times, etc) that shows an operation (i.e. It tells us what to do with the value(s).
Operators take a function as an input and give a function as an output. It tells us what to do with the value(s). An operator is a symbol, like +, ×, etc, that shows an operation. A term is either a single number or a. The difference between an operator and a function is simply that we've decided to call the operator an operator and we've decided to. A mapping of one set into another, each of which has a certain structure (defined by algebraic operations, a topology, or by an order. As an example, consider $\omega$, an operator on the set of functions. A symbol (such as , minus, times, etc) that shows an operation (i.e.
An operator is a symbol, like +, ×, etc, that shows an operation. A mapping of one set into another, each of which has a certain structure (defined by algebraic operations, a topology, or by an order. A term is either a single number or a. A symbol (such as , minus, times, etc) that shows an operation (i.e. Operators take a function as an input and give a function as an output. It tells us what to do with the value(s). As an example, consider $\omega$, an operator on the set of functions. The difference between an operator and a function is simply that we've decided to call the operator an operator and we've decided to.
"Nabla operator definition, math and calculus basics dark version
As an example, consider $\omega$, an operator on the set of functions. Operators take a function as an input and give a function as an output. The difference between an operator and a function is simply that we've decided to call the operator an operator and we've decided to. An operator is a symbol, like +, ×, etc, that shows.
"Nabla Operator Definition Math And Calculus Basics" Sticker for Sale
The difference between an operator and a function is simply that we've decided to call the operator an operator and we've decided to. Operators take a function as an input and give a function as an output. A mapping of one set into another, each of which has a certain structure (defined by algebraic operations, a topology, or by an.
"Nabla operator definition, math and physics basics" Sticker for Sale
As an example, consider $\omega$, an operator on the set of functions. A symbol (such as , minus, times, etc) that shows an operation (i.e. A mapping of one set into another, each of which has a certain structure (defined by algebraic operations, a topology, or by an order. A term is either a single number or a. Operators take.
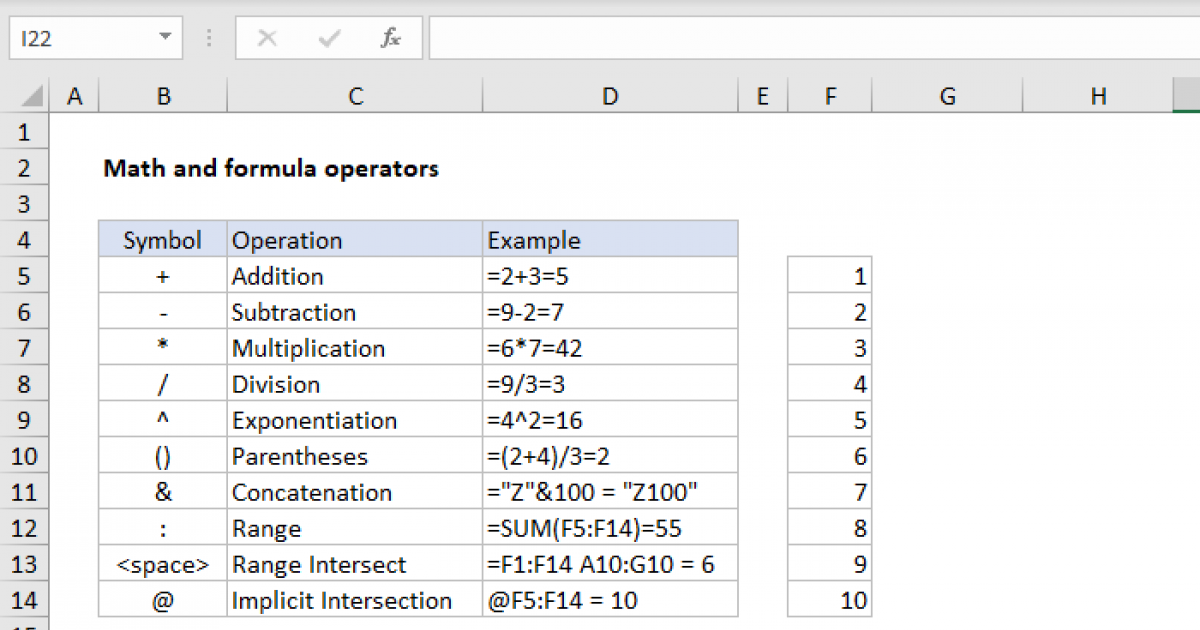
Excel Math Operators Exceljet
The difference between an operator and a function is simply that we've decided to call the operator an operator and we've decided to. An operator is a symbol, like +, ×, etc, that shows an operation. A term is either a single number or a. As an example, consider $\omega$, an operator on the set of functions. It tells us.
"Nabla Operator Definition Math And Calculus Basics" Poster for Sale
As an example, consider $\omega$, an operator on the set of functions. The difference between an operator and a function is simply that we've decided to call the operator an operator and we've decided to. A mapping of one set into another, each of which has a certain structure (defined by algebraic operations, a topology, or by an order. It.
Nabla operator definition, linear algebra and math Math Pin TeePublic
It tells us what to do with the value(s). An operator is a symbol, like +, ×, etc, that shows an operation. A term is either a single number or a. A symbol (such as , minus, times, etc) that shows an operation (i.e. As an example, consider $\omega$, an operator on the set of functions.
Nabla operator definition, differential calculus and math Math
A mapping of one set into another, each of which has a certain structure (defined by algebraic operations, a topology, or by an order. A term is either a single number or a. The difference between an operator and a function is simply that we've decided to call the operator an operator and we've decided to. Operators take a function.
Nabla operator definition, linear algebra and math Math Posters and
A symbol (such as , minus, times, etc) that shows an operation (i.e. A term is either a single number or a. The difference between an operator and a function is simply that we've decided to call the operator an operator and we've decided to. An operator is a symbol, like +, ×, etc, that shows an operation. A mapping.
"Nabla operator definition, math and calculus basics dark version" Art
A symbol (such as , minus, times, etc) that shows an operation (i.e. It tells us what to do with the value(s). A term is either a single number or a. Operators take a function as an input and give a function as an output. The difference between an operator and a function is simply that we've decided to call.
"Nabla Operator Definition Math And Calculus Basics" Poster for Sale
A term is either a single number or a. A mapping of one set into another, each of which has a certain structure (defined by algebraic operations, a topology, or by an order. As an example, consider $\omega$, an operator on the set of functions. A symbol (such as , minus, times, etc) that shows an operation (i.e. Operators take.
It Tells Us What To Do With The Value(S).
The difference between an operator and a function is simply that we've decided to call the operator an operator and we've decided to. A mapping of one set into another, each of which has a certain structure (defined by algebraic operations, a topology, or by an order. Operators take a function as an input and give a function as an output. A term is either a single number or a.
A Symbol (Such As , Minus, Times, Etc) That Shows An Operation (I.e.
As an example, consider $\omega$, an operator on the set of functions. An operator is a symbol, like +, ×, etc, that shows an operation.