Moment Generating Function Of A Binomial Distribution
Moment Generating Function Of A Binomial Distribution - Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. Moment generating functions definition 2.3.6. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf.
Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. Moment generating functions definition 2.3.6. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf.
The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
Binomial Distribution Derivation of Mean, Variance & Moment
Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf.
PPT Moment Generating Functions PowerPoint Presentation, free
The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
Moment Generating Functions 8 MGF of binomial mean YouTube
Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. Moment generating functions definition 2.3.6. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf.
.jpg)
Moment Generating Functions ppt download
Moment generating functions definition 2.3.6. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
Negative binomial distribution
Moment generating functions definition 2.3.6. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
PPT Moment Generating Functions PowerPoint Presentation, free
Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions definition 2.3.6.
Negative binomial moment generating function YouTube
The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. Moment generating functions definition 2.3.6.
![[Math] Deriving the moment generating function of the negative binomial](https://i.stack.imgur.com/EGd8a.jpg)
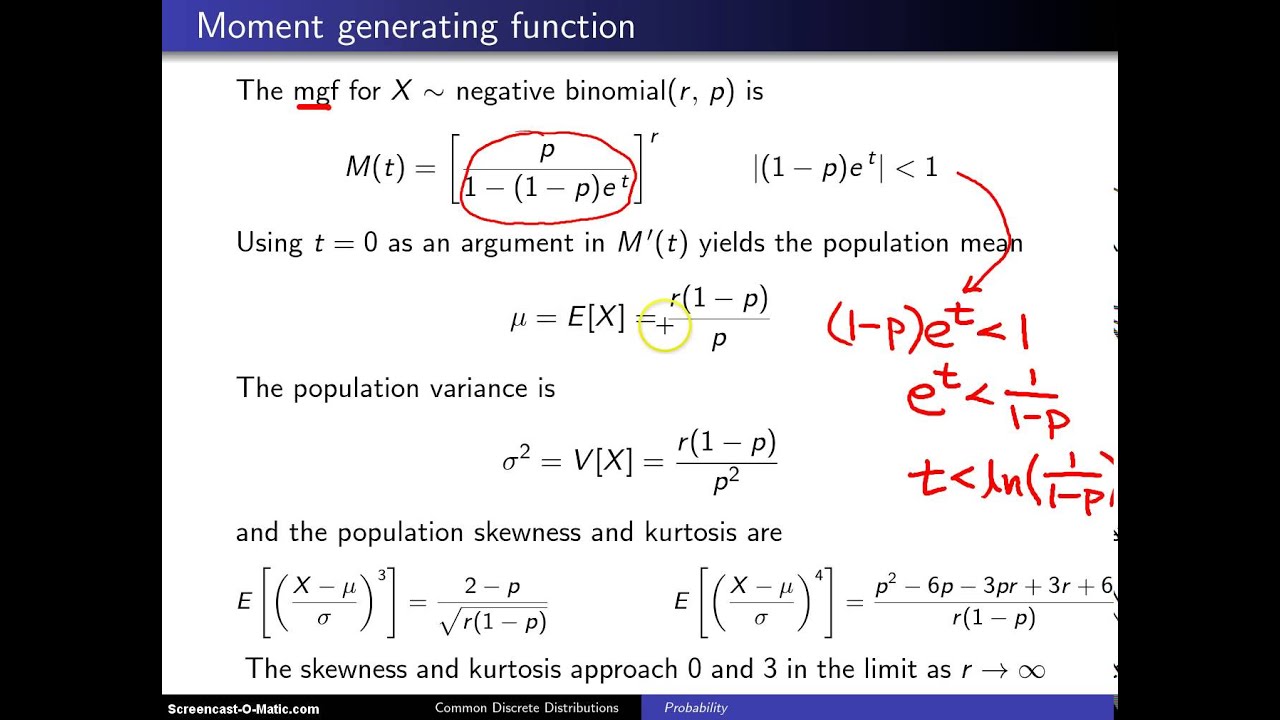
[Math] Deriving the moment generating function of the negative binomial
The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. Moment generating functions definition 2.3.6.
What is Moment Generating Functions (MGF)?
Moment generating functions definition 2.3.6. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf.
SOLUTION NU Math 206 Lecture Moment generating function Bernoulli
Moment generating functions definition 2.3.6. The moment generating function (mgf) of a random variable x is mx(t) = e(etx) = (åx e txf. Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient.
The Moment Generating Function (Mgf) Of A Random Variable X Is Mx(T) = E(Etx) = (Åx E Txf.
Moment generating functions (mgfs) are an essential tool in probability and statistics, providing a compact and efficient. Moment generating functions definition 2.3.6.